POLYNOMIAL || REMAINDER THEOREM || Fully Explained with Example ||
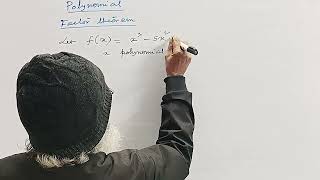
21:31
POLYNOMIAL || FACTOR THEOREM || Factorise (x^3- 6x^2+ 11x - 6) With the help of Factorise Theorem

16:50
y= (tanx)^x+e^sinx Then (dy/dx) =e^(sinx)cos x +( tanx)^x [ log(tanx)+ x secx cosecx]
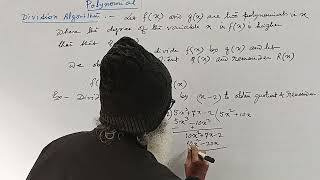
15:59
POLYNOMIAL || DIVISION ALGORITHM || Fully Explained With Example ||

22:54
PRINCIPAL VALUE of arc cos ( - 1/√2 ) || PRINCIPAL VALUE || INVERSE TRIGONOMETRIC FUNCTIONS
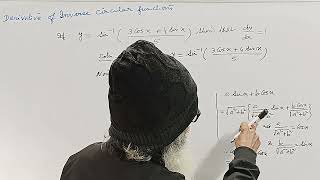
12:49
y= arc sin {(3cosx + 4sinx) /5} Then ( dy/ dx) = 1 || DERIVATIVE | INVERSE CIRCULAR FUNCTIONS

12:24
POLYNOMIAL || (x^3 +3x^2- Kx +4 ) is divisible by ( x- 2) || Value of K || Remainder Theorem ||
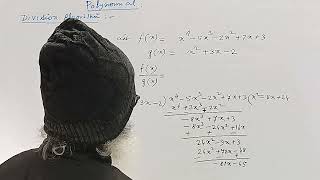
13:28
POLYNOMIAL || DIVISION of TWO POLYNOMIALS May or may not be a POLYNOMIAL || Explained With Example

12:19