POLYNOMIAL || REMAINDER THEOREM || Fully Explained with Example ||
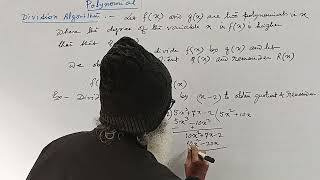
15:59
POLYNOMIAL || DIVISION ALGORITHM || Fully Explained With Example ||

15:58
arc tan [(1/√3) tan( x/2) ]=(1/2) arc cos {(1+2cosx) /( 2+cosx) }

15:53
Value of arc sin (sin10) || Concept of General solution of Trigonometric Equation ||
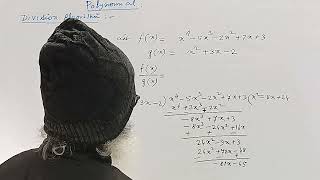
13:28
POLYNOMIAL || DIVISION of TWO POLYNOMIALS May or may not be a POLYNOMIAL || Explained With Example

18:00
y= √{t- A) /(B- t)} and x= √{( t- A)(B- t)} Then (dy/dx) = ? |DIFFERENTIATION | Special Substitution

16:50
y= (tanx)^x+e^sinx Then (dy/dx) =e^(sinx)cos x +( tanx)^x [ log(tanx)+ x secx cosecx]

10:59
A POLYNOMIAL f(x) be DIVIDED by (ax+b) , 'a' not equal to 0 || REMAINDER will be f(- b/a) || EXAMPLE

15:42