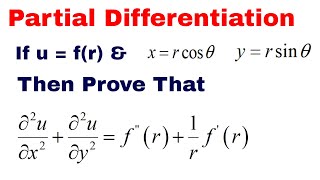
If u=f(r) where r^2=x^2+y^2, show that ∂^2u/∂x^2+∂^2u/∂y^2=f''(r)+1/r f'(r)| Partial Differentiation

11:30
If u = log(x^3 + y^3 + z^3 − 3xyz) then (∂^2u/∂x^2+∂^2u/∂y^2+∂^2u/∂z^2)= −3/(x + y + z)2

15:34
Partial Differentiation #8 in Hindi (M.imp) | Engineering Mathematics

17:04
If u=f(r) where r2=x2+y2+z2 then dx2d2u+dy2d2u+dz2d2u=f"(r)+r4f′(r) partial differentiation
12:27
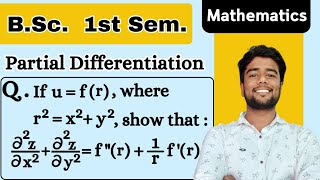
If u=f(r), where r^2 = x^2 + y^2 , show that d^2 z/dx^2 +d^2 z/dy^2 = f"(r)+1/r f'(r)
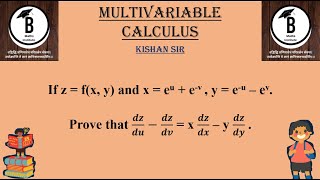
8:06
If z = f(x, y) and x = eu + e-v , y = e-u – ev. Prove that 𝒅𝒛/𝒅𝒖 − 𝒅𝒛/𝒅𝒗 = x 𝒅𝒛/𝒅𝒙 – y 𝒅𝒛/𝒅𝒚 .
11:48
'My jaw is dropped': Canadian official's interview stuns Amanpour
10:48
Partial Differentiation Problem- 3
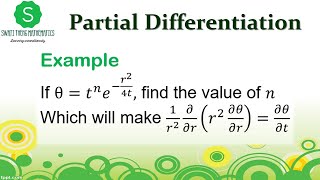
14:21