Group theory, prove that every cyclic group is abelian

5:30
Group theory, if every non- identity element of a group has order 2 then G is an abelian group

11:42
Group theory, prove that G = ( 1, -1, i, -i ) forms an abelian group under multiplication

5:01
Cyclic Groups (Abstract Algebra)

5:41
Group Theory: Proof of the Formula for the Inverse of a Product
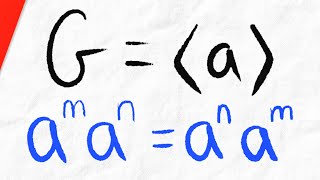
3:19
Every Cyclic Group is Abelian | Abstract Algebra

12:17
Show that G = { a + √3b : a,b ∈ Q } is an abelian group under addition of real numbers.

9:35
301.7C When are Cosets of H also Subgroups?

25:55