4:17

Dihedral Group (Abstract Algebra)

5:01

Cyclic Groups (Abstract Algebra)

5:30

Symmetric Groups (Abstract Algebra)

11:15

Group Definition (expanded) - Abstract Algebra

13:21

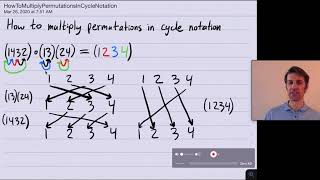
Abstract Algebra. How to multiply permutations in cycle notation

18:24

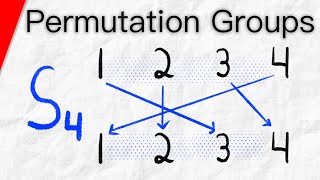
Permutation Groups and Symmetric Groups | Abstract Algebra

9:19

Cosets and Lagrange’s Theorem - The Size of Subgroups (Abstract Algebra)

8:55

Direct Products of Groups (Abstract Algebra)