A Wallis-type product for e.

17:27
e is golden.

19:30
a notorious functional equation.
26:38
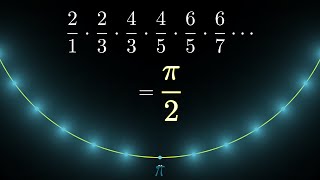
The Wallis product for pi, proved geometrically

24:21
How to integrate with respect to any function!

8:24
|i Factorial| You Won't Believe The Outcome
14:38
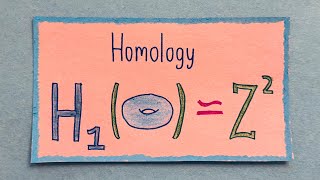
What is algebraic topology?

19:37
a twist on a classic improper integral

10:42