A line s drawn through a variable point on the ellipse x2/a2+y2/b2=1,z=0 to meet two fixed line y=mx

26:28
prove that the locus of a line which meets the lines y=mx, z=c, y=-mx , z=-cand the circle x2+y2=a2

24:08
find the constant a,b,c so that F=(x+2y+az)i+(bx-3y-z)j+(4x+cy+2z)k is irrotational

13:30
using complex variable, evaluate the real integral

17:08
show that A=(6xy+z3)i+(3x2-z)j+(3xz2-y)k is irrotational. find Φ such that A=∇Φ
20:01
7 January 2025

40:14
Los misterios del # 7
12:25
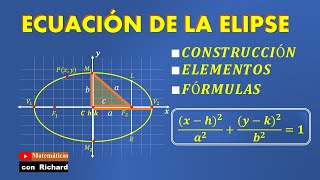
Ecuación de la elipse
12:16