Integration by (u-)substitution
30:31
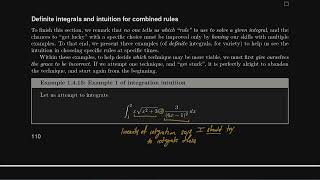
Intuition for integration techniques
31:20
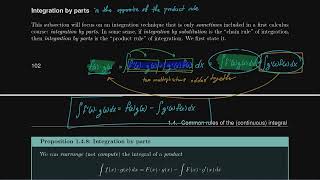
Integration by parts
25:48
How to Integrate Using U-Substitution (NancyPi)

27:57
Arithmetic and operations
29:40
The (continuous) derivative

20:37
Integrate x^-x dx

16:59
integration by parts, DI method, VERY EASY

32:12